Henon Map Maximum Lyapunov Exponent and
Kaplan-Yorke Dimension
J. C. Sprott
Department of Physics, University of
Wisconsin,
Madison, WI 53706, USA
March 13, 2007
The Henon map is a prototypical 2-D invertible dissipative
iterated map with
chaotic
solutions proposed by the French astronomer Michel Henon (M. Henon,
Commun.
Math. Phys. Phys. 50, 69-77 (1976)) as a simplified model of
the
Poincare map for the Lorenz model:
xn+1 = 1 - axn2
+ byn
yn+1 = xn
Since the second equation above can be written as yn
= xn-1, the Henon map can be written in
terms
of a single variable with two time delays:
xn+1 = 1 - axn2
+ bxn-1
An interesting question to ask is what are the values of a and b that maximize the largest
Lyapunov exponent and the Kaplan-Yorke
dimension. The maximum Lyapunov exponent occurs for a = 2 and b = 0, where the map reduces to a
one-dimensional quadratic map whose largest Lyapunov exponent is ln(2)
= 0.693147181... . The other exponent is minus infinity, which implies
that there is infinitely rapid contraction in the direction
perpendicular to the one-dimensional parabolic attractor.
Much less trivial is the question of what values of a and b maximize the Kaplan-Yorke
dimension. The area contraction for the Henon map is the sum of the two
Lyapunov exponents and is given by ln(|b|)
which is negative (contractive) for -1 < b < 1 and encompasses the entire
region over which the map is bounded as well as chaotic. From the
largest Lyapunov exponent L,
the Kaplan-Yorke dimension is therefore given by Dky = 1 + L/(L
- ln|b|).
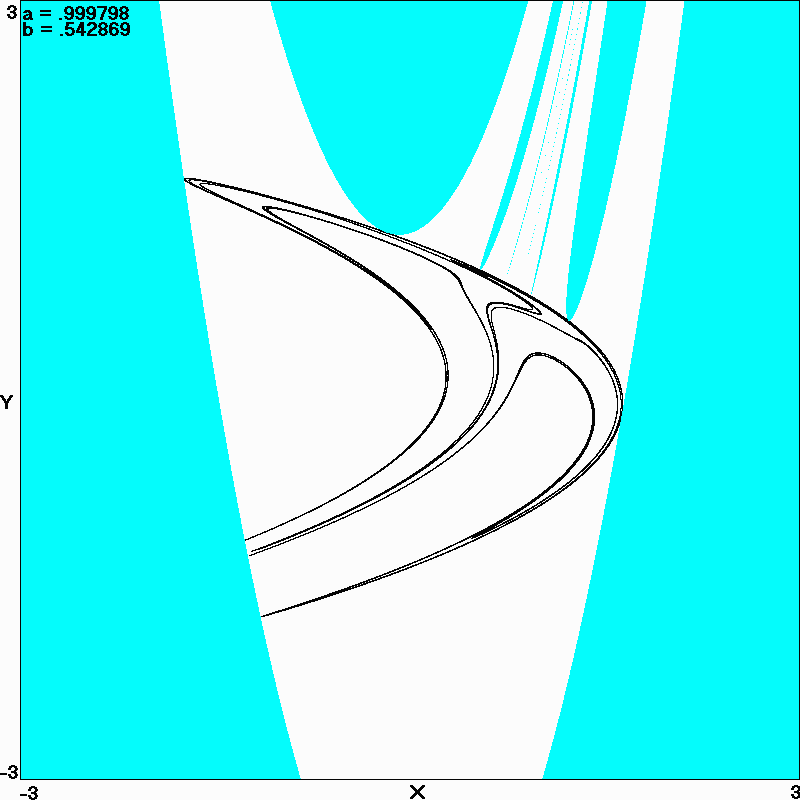
A program was written to search ab-plane for the maximum value of Dky giving a result that
is suspiciously close to a =
1.0 with b = 0.5427 for which
L = 0.2716 and Dky = 1.3076. The
attractor for this case along with its basin of attraction (in white)
is shown below:
Note that the attractor nearly touches its basin boundary in numerous
places, which is typical for a chaotic system where the maximum chaos
often occurs just before the orbit becomes unbounded. Note also that
the basin boundary has numerous (perhaps infinitely many) narrow
tongues, suggesting a possibly fractal structure that deserves further
study. The attractor is not greatly different from the Henon map with the usual parameters of a = 1.4 and b = 0.3 for which L = 0.419222 and Dky = 1.258269 (see Sprott). Thus the usual parameters are close to
those for which the attractor dimension is maximized, and all of the
attractors are distinctly "Henon-like."
Back to Sprott's Technical Notes