Elementary quadratic chaotic flows with no equilibria

Sajad Jafaria,∗, J.C. Sprottb, S. Mohammad Reza Hashemi Golpayegania
aBiomedical Engineering Department, Amirkabir University of Technology, Tehran 15875-4413, Iran
bDepartment of Physics, University of Wisconsin, Madison, WI 53706, USA

Received 23 October 2012
Received in revised form 5 January 2013
Accepted 7 January 2013
Available online 16 January 2013
Communicated by C.R. Doering

Three methods are used to produce a catalog of seventeen elementary three-dimensional chaotic flows with quadratic nonlinearities that have the unusual feature of lacking any equilibrium points. It is likely that most if not all the elementary examples of such systems have now been identified.

Ref: S. Jafari, J. C. Sprott, and S. M. R. H. Golpayegani, Phys. Lett. A 377, 699-702 (2013)

The complete paper is available in PDF format.

Return to Sprott's Books and Publications.


Fig. 1. State space diagram of the cases in Table 1 projected onto the xy-plane
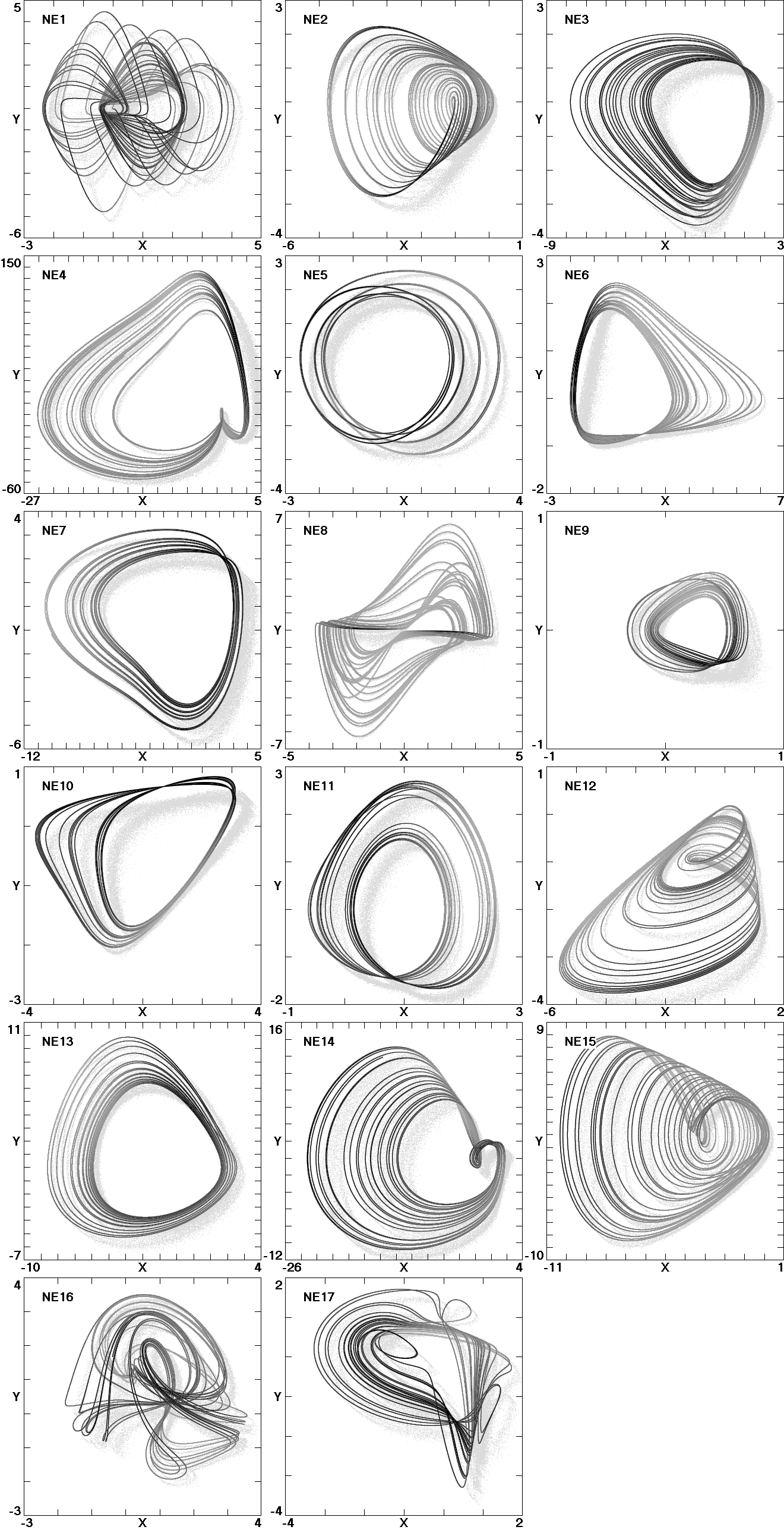
Figure 1

Fig. 2. The largest Lyapunov exponent and bifurcation diagram of NE6 showing a period-doubling route to chaos.
Figure 2