Simplifications of the Lorenz Attractor

J. C. Sprott
University of Wisconsin, Madison

ABSTRACT

The Lorenz attractor was once thought to be the mathematically simplest autonomous dissipative chaotic flow, but it is now known that it is only one member of a very large family of such systems, many of which are even simpler. Even the system originally proposed by Lorenz is not in its simplest possible form. This paper will describe a number of simplifications that can be made to the Lorenz system that preserve its dynamics as well as a number of chaotic systems that are much simpler and hence can serve as alternate models of chaos.

Ref: J. C. Sprott,  Nonlinear Dynamics, Psychology, and Life Sciences 13, 271-278  (2009).

The complete paper is available in PDF format.

Return to Sprott's Books and Publications.

Figure 1

Fig. 1. The Lorenz attractor

Figure 2

Fig. 2. Simplified variants of the Lorenz attractor

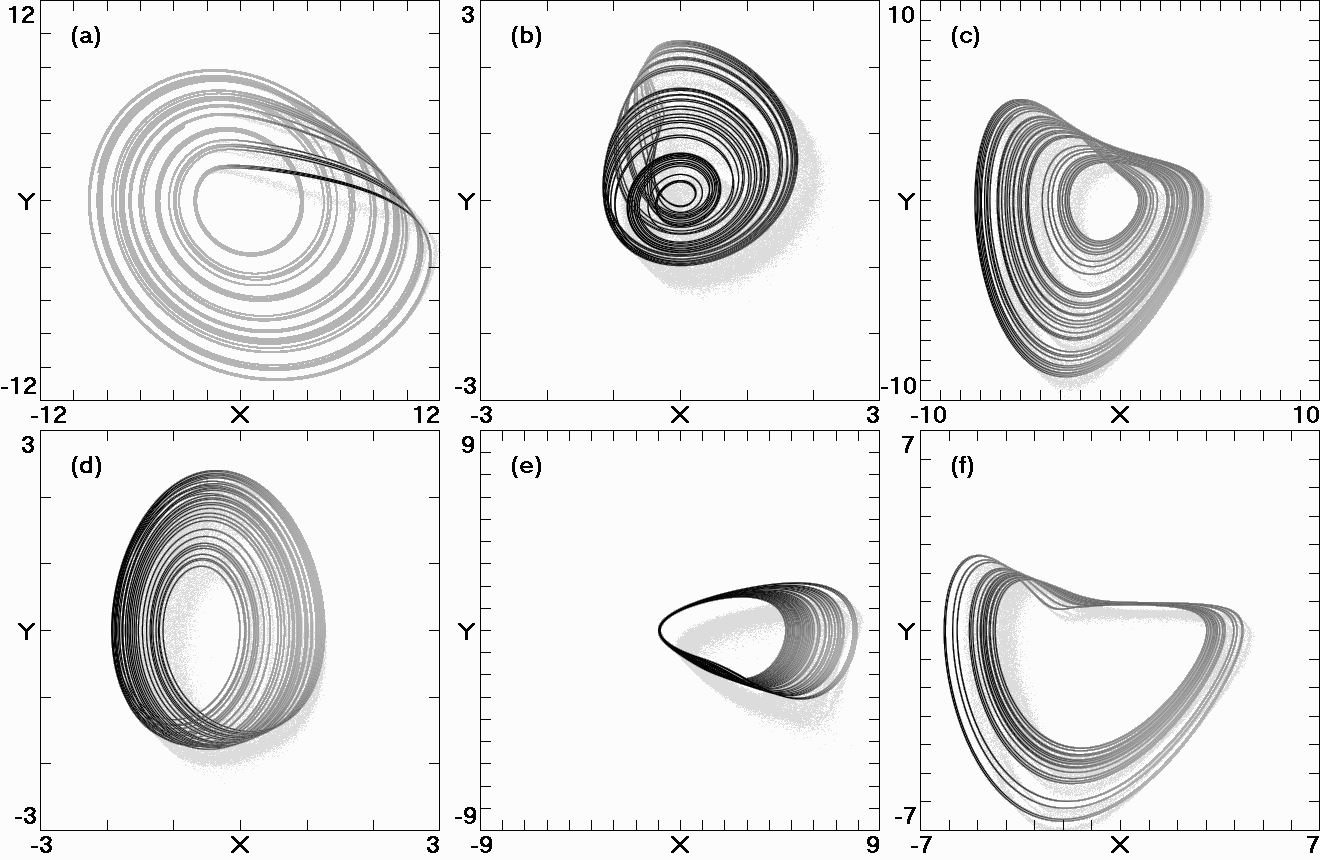
Figure 3

Fig. 3. Folded bands

Figure 4

Fig. 4. Jerky Lorenz-like systems