December 21, 1998 Physics 207
Final Exam
Print your name and section clearly on all nine pages. (If you do not
know your section number, write your TA’s name.) Show all work in the space
immediately below each problem. Your final answer must be placed in the
box provided. Problems will be graded on reasoning and intermediate steps
as well as on the final answer. Be sure to include units wherever necessary,
and the direction of vectors. Each problem is worth 25 points. In doing
the problems, try to be neat. Check your answers to see that they have
the correct dimensions (units) and are the right order of magnitudes. You
are allowed two 8½ x 11" sheets of notes and no other references.
The exam lasts exactly 2 hours.
(Do not write below)
SCORE:
Problem 1: __________
Problem 2: __________
Problem 3: __________
Problem 4: __________
Problem 5: __________
Problem 6: __________
Problem 7: __________
Problem 8: __________
TOTAL: ___________
First Name: ___________________ Last Name: ____________________ Section: _________
1. A target is located 30 meters horizontally and 10 meters above you. You throw a ball toward the target at an angle of 45º from the horizontal.
a. With what speed must you throw the ball in order to hit the target?
(15
pts.)
b. For how much time is the ball in flight before it hits the target?
(10
pts.)
First Name: ___________________ Last Name: ____________________ Section: _________
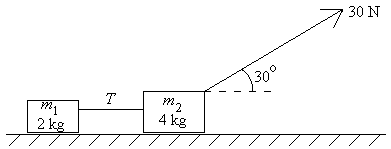
2. Two blocks connected by a massless string slide across a horizontal floor with a coefficient of kinetic friction m = 0.4 in response to a 30-N force as shown.

b. What is the tension T in the string connecting the blocks?(10
pts.)
First Name: ___________________ Last Name: ____________________ Section: _________
3. A bicyclist rides at a speed of 10 km/hr up a hill that rises 60 m in a distance of 1 km. Assume the bicycle plus person has a mass of 80 kg. Neglect energy losses due to friction.
a. How much work does the bicyclist do? (5 pts.)
b. What power does the bicyclist produce? (5 pts.)
c. If the pedals rotate once per second on a diameter of 36 cm, what
average tangential force must the bicyclist produce on the pedals? (5
pts.)
d. For the conditions above, what average torque is applied to the pedals?
(5
pts.)
e. What minimum coefficient of friction between the wheels and the ground
is required to keep the wheels from slipping? (5 pts.)
First Name: ___________________ Last Name: ____________________ Section: _________
4. Suppose all 5 x 109 human inhabitants of the Earth line up on the equator and begin walking westward at a speed of 2 m/s.
a. If the average mass of a person is 70 kg, what impulse is applied
to the Earth? (5 pts.)
b. If the Earth is a uniform sphere with mass M = 6 x 1024
kg, radius R = 6000 km, and moment of inertia I = 0.4MR2,
by what fraction would its rotational velocity change? (10 pts.)
c. Now imagine that all the inhabitants of the Earth crowded together
in a single location and simultaneously jumped upward, reaching an average
height of 0.5 m. What velocity is imparted to the Earth? (10 pts.)
First Name: ___________________ Last Name: ____________________ Section: _________
5. A meter stick with a density of 700 kg/m3 is lowered end on into a swimming pool filled with water.
a. How much of the meter stick is above the water when the meter stick
reaches static equilibrium with the water? (8 pts.)
b. If the meter stick is raised 10 cm above its equilibrium position
and released, with what period does it oscillate? (10 pts.)
c. For the condition above, what is the maximum speed of oscillation
of the meter stick? (7 pts.)
First Name: ___________________ Last Name: ____________________ Section: _________
6. In a search for planets orbiting distant stars, you observe a star 40 light years away (3.8 x 1017 m) that wobbles with a period of 80 (earth) days and an amplitude of 3 x 10-8 degrees. Assume the star has the same mass as the sun (1.99 x 1030 kg).
a. What is the distance of the planet from the star assuming it is in
a circular orbit? (6 pts.)
b. What is the mass of the planet? (7 pts.)
c. If the intensity of heat radiation from the star reaching the Earth
is 2 x 10-10 W/m2, what is the intensity of heat
radiation at the orbit of the planet? (6 pts.)
d. What is the average surface temperature of the planet in degrees
Celsius? (6 pts.)
First Name: ___________________ Last Name: ____________________ Section: _________
7. A violin string with a mass of 0.5 g and a length of 35 cm produces a fundamental frequency of 440 Hz (concert A) when bowed.
a. What is the tension in the string? (7 pts.)
b. If an observer moves toward the violin at a speed of 20 m/s, what frequency is heard?
(6 pts.)
c. If the tension in the string is increased by 1%, what beat frequency
is heard by an observer at rest when the violin is played simultaneously
with another violin that is in tune? (6 pts.)
d. If the sound intensity is 60 dB at a distance of 5 m from the violin,
what acoustical power does the violin produce, assuming it radiates equally
in all directions? (6 pts.)
First Name: ___________________ Last Name: ____________________ Section: _________
8. A 100-g ice cube at 0° C is mixed with 200 cm3 of water at 70° C and allowed to reach thermal equilibrium in a thermally insulated container.
a. What is the final temperature of the mixture? (6 pts.)
b. What is the change in entropy of the mixture? (7 pts.)
c. How many joules of energy must be added to the mixture to heat it
to 100° C and vaporize it? (6 pts.)
d. Assuming the water vapor at 100° C
is an ideal gas with a density of 18 g/mole, what volume will it occupy
at a pressure of 1 x 105 Pa? (6 pts.)